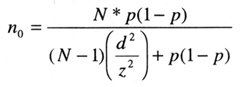
January, 2000, vol.11 no.1
H.-S. Han and L.D. Kellogg
University of Northern British Columbia
Prince George, BC, Canada
and
Oregon State University
Corvallis, Oregon, USA
ABSTRACT
Four sampling methods were compared for accuracy and ease of implementation in measuring residual stand damage. Data were collected from young Douglas-fir (Pseudotsuga menziesii) stands, which were commercially thinned using three different logging systems in western Oregon. Systematic plot sampling consistently provided damage estimates similar to the results of a 100% survey; there was no significant difference between their accuracies in measuring stand damage. This method also took the least amount of time and effort for map layout and field plot location. Because measuring stand damage requires considerable effort in sample planning and implementation, an easier, quick-survey method should be developed to monitor residual stand damage for in-progress and post-thinning operations.
Keywords: timber harvesting systems, partial cutting, young stand management, logging injury.
The authors are. respectively, Assistant Professor, Forestry Program, College of Science and Management, and Professor, Department of Forest Engineering, College of Forestry.
INTRODUCTION
As thinning becomes more widely practiced on both public and private forestlands, the containment of residual stand damage is more critical for landowners and logging contractors. Excessive wounding of the remaining trees may greatly reduce the expected thinning benefits, such as greater tree vigor and increased size. In western Oregon, a timber sale administrator can stop logging operations at any time if damage to residual trees is beyond the tolerable level. Penalties can also be assessed for excessive logging damage. For example, if a logging contractor damages more than 5% of the remaining trees (scar size per tree greater than 155 cm2 [24 in.2]) on a timber sale on Oregon Department of Forestry land, the contractor can be fined up to twice the contract value for each damaged tree, depending on severity [14]. The penalty can be even more severe on private lands, where causing excessive damage can deny one the chance of gaining another contract with the same landowner.
Because it is not feasible to check every tree in a large area, sampling to estimate stand damage levels has been used by forest managers and researchers. Past studies (Table 1) have used different sampling patterns or plot sizes that may result in wide variations in damage estimates. The most popular methods are systematic plot and simple random plot sampling. These methods use a fixed-radius or variable-radius plot, or a rectangular block. Systematic transects and block sampling can also be used. transects between boundaries eliminate the variability in the location of damaged trees some distance from the corridor. Blocks can be installed in relation to skid trails or skyline roads, where most logging damage occurs.
Because damage is concentrated along skid trails or skyline corridors, the entire stand can be stratified into two areas, the trail and off-trail strata, then sampled by different methods. Stratified sampling reduced the standard deviation of the estimates relative to an unstratified, simple random design, but the strategy required traversing the entire skid trail system and setting up the boundaries between two areas [17].
One of the challenges in measuring stand damage is adhering to a complicated sampling plan, which may take a week or more to implement. Walking over logging slash is not safe and it is even more arduous to follow a precise azimuth and slope distance. Supervising in-progress thinning operations is difficult if a landowner or forest manager lacks information on tree conditions. An efficient survey method is needed to facilitate assessment of stand damage.
In this study, an optimal sampling method was determined by comparing damage estimates from four conventional sampling methods with the actual damage level derived from a 100% survey. Ease of field implementation was also considered. The sampling methods included 1) systematic plot sampling, 2) random plot sampling, 3) systematic transect, and 4) block along a skyline corridor/skid trail. The concept of an easy, quick survey was also proposed for monitoring stand damage levels for in-progress or post-thinning operations, without requiring a detailed sampling procedure. It presented in the results and Discussion section.
TABLE 1. Selected sampling methods used in past studies
| Study | Logging system | Sampling method | Plot type | Plot size (ha [ac]) |
| Nyland and Gabriel (1972)[13] |
tractor- skidder | Systematic | Variable-radius | Various |
| Burditt (1981)[3] | Cable | Systematic | Fixed-radius | 0.08 [0.20] |
| Caccavano (1982)[4] | Cable | Systematic | transect | Various |
| Aho et al. (1983)[1] | tractor | Systematic | Fixed-radius | 0.01 [0.02] |
| Kelly (1983)[10] | Feller-Buncher- Skidder | Random | Fiexed-radius | 0.08 [0.20] |
| Ostrofsky et al. (1986)[15] |
Feller-Buncher Chainsaw- Skidder | Random | Block | 0.04 or 0.08 [0.10 or 0.20] |
| Sidle and Laurent (1986)[16] |
Walking Backhoe | Block along skid trails | Block | Various |
| Cline et al. (1991)[5] | Feller-Buncher -Skidder | Block along skid trails | Block | Various |
| Fairweather (1991)[6] | Cable | Random | Fixed-radius, Variable-radius |
0.02 [0.05] and Various |
| Flatten (1991)[7] | Helicopter | Systematic | Fixed-radius | 0.20 [0.50] |
| McLaughlin and Pulkki (1992)[12] |
Chainsaw -Forwarder Harvester -Skidder | Random | Fixed-radius | 0.04 [0.10] |
| Bettinger and Kellogg (1193)[2] |
Cut-to-length | Random | Fixed-radius | 0.04 [0.10] |
| Lanford and Stokes (1195)[11] |
Skidder Cut-to-Length | Random | Fixed-Radius | 0.01 [0.02] |
METHODS
Study Site Descriptions
Data were collected on commercially thinned young stands on the Siuslaw and Willamette National Forests (NF) in western Oregon (Table 2). The three units in the Siuslaw NF were located near Yachats in the Coast Range. Species consisted of predominantly Douglas-fir (Pseudotsuga menziesii), and others such as western hemlock (Tsuga heterophylla) and Sitka spruce (Picea sitchensis). In the Willamette NF, three units were selected: Walk Thin and Flat Thin in the Oakridge Ranger District, and Mill Thin in the Blue River Ranger District. Stands were dominated by Douglas-fir, with scattered western hemlock and bigleaf maple (Acer macro-phyllum). The mean dbh for trees at all sites are about the same, 25.4 - 30.5 cm [10 - 12 in.], but trees in the Siuslaw NF were younger (mean age: 34) than those in the Willamette NF (mean age: 43 - 46).
trees in the Yachats and Walk Thin units were thinned with a small yarder, a Koller 501. Skyline corridors averaged 3.6 m [12 ft] wide, spaced 45 m [150 ft] apart. Tailtrees were rigged on 100% and 84% of the skyline roads in Yachats and Walk Thin, respectively. The skyline corridors at Yachats were arranged in a fan-shaped pattern from three landings, with intermediate supports on 38% of skyline roads. Walk Thin had a predominantly parallel-corridor pattern. The tractors at Mill Thin were CASE 550 and D-5 CAT crawlers. The cut-to-length system at Flat Thin included a Timberjack 2518 carrier with a Waterous 762b hydraulic single-grip harvesting head, and an FMG 910 forwarder. Skid trail width averaged 3.6 m [12 ft]. Spacing between trails ranged from 36.4 m [120 ft] for tractor skidding to 18.2 m [60 ft] for the cut-to-length thinning. The skidding pattern was a branching type for tractor logging, but was parallel for cut-to-length operations.
TABLE 2. Study sites and stand descriptionabefore commercial thinning
| Before Thinning | |||||||||
| Site/ unit |
Loggin system |
Thinning prescription (remaining tph[tpa])b |
Study area (ha [ac]) |
Mean age (yr) |
Mean dbh (cm {in.]) |
Mean ht (m [ft]) |
tph [tpa]b |
Basal area (m2/ha) [ft2/ac] |
% Slope |
| Siuslaw Yachats |
Skyline | 247 [100] 148 [60] |
5.3 [13] 3.6 [9] |
34 | 26.9 [10.6] |
23 [75] |
790 [320] |
48.4 [210.6] |
12-70 |
| Willamette Walk Thin |
Skyline | 284 [115] | 4.0 [9.8] |
45 | 26.4 [10.4] |
22 [74] |
667 [270] |
27.1 [118] |
5-80 |
| Mill Thin | tractor | 284 [115] | 4.3 [10.5] |
43 | 30.0 [11.8] |
24 [78] |
573 [232] |
39.5 [172] |
0-15 |
| Flat Thin | Cut-to Length |
284 [115] | 3.0 [7.4] |
45 | 28.7 [11.3] |
23 [77] |
504 [204] |
42.7 [186] |
0-20 |
Data Collection
During the summers of 1995 and 1996 and March of 1997, every tree in the six units was checked. If a tree was wounded, it was numbered with fluorescent paint on all sides for easy identification during the four different sampling surveys. Damage data and dbh measurement for each damaged tree were related to this identification number and stored in a spreadsheet program file. Three damage types were recorded: scarring larger than 6.5 cm2 [1 in.2]; crown removal greater than 50%, including broken-top; and obvious scarring or severing of root systems. trees leaning more than 10o from vertical also were considered damaged.
After the 100% survey, the experimental sample size of each unit was determined, based on Thompson�s calculation of the number of damaged trees needed for sampling [18].
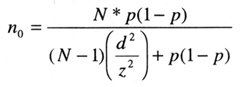
Sample size for systematic plot and random plot sampling
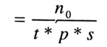
where:
n0 = number of damaged trees required in sample.
N = total number of trees in the unit. For this study,
we counted all the trees in each unit; N can also be estimated by multiplying
unit area by target number of trees to be left.
p = estimate of percent damaged trees in unit. The
formula depends on the unknown population proportion p. If no estimate
of p is available before the survey, a �worst case� value of p = 0.5 can
be used to determine sample size.
d = width of the confidence interval, 10% in this study
(d = 0.10).
z = the upper α/2 point of the normal distribution (1.96
for 95% probability, α=0.05)
t = the estimated number of trees per ha (or trees per
ac).
s = fixed-radius circular plot size, 0.04 ha [0.10 ac]
or 0.08 ha [0.20 ac].
Sampling Methods Studied
Once the sample size was determined, we tested four sampling methods for the accuracy of their estimates and simplicity in field performances. The sampling layout for each method is shown in Figure 1. For purposes of comparison, the same value of n0 was used for all methods. All the trees within a sampling plot, transect, or block were counted, and damage data were transferred for each numbered tree, as collected in the 100% survey. The standard error (Sx) and confidence intervals for damage estimates by the sampling methods were computed. Standard errors were used for Z-tests to compare estimates from four sampling methods to the actual damage seen in the 100% survey. The values from the Z-test were used to compute a p-value for each estimate. Any comments (pro and con) about implementing the sample method at each site were also recorded.
Systematic plot sampling
In mapping this method, we drew lines perpendicular to the primary direction of the skyline corridors or skid trails. This avoided paralleling the corridors or trails where most of the damaged trees are concentrated [2]. Sampling plots were systematically installed over the unit by applying an interval of constant length after a random start. The line interval was wider than the interval between plots. Data were collected from all residual trees on 20 to 27 fixed-radius plots, depending on the damage level previously determined by the 100% survey. Plot size was 0.04 ha [0.10 ac] for all units except for the unit with 74 trees per ha (tph) [30 trees per ac (tpa)] at Yachats, where a 0.08-ha [0.20-ac] plot was needed to obtain an adequate number of trees.
Random plot sampling
The sample and plot sizes used in random plot sampling were the same as in the systematic method for each unit. In order to install plots, a grid was laid on a unit map and sets of x and y coordinates were randomly generated using a spreadsheet program. Plots were located by these coordinates within the unit boundaries. If a plot overlapped a unit boundary or another plot, this plot was deleted and replaced with another. A traversePC [8] program was used to generate a set of azimuths and horizontal distances between plots. To minimize traveling distances and walking up- or downhill, we listed plots in order before entering coordinates into traversePC.

Display large image of Figure 1
Figure 1. Layout of sampling methods
Systematic transect
The same lines used in systematic plot sampling served as transect centerlines. The width of a transect was determined from the total line length on the map and by the number of required damaged trees, n0. The width increased as n0 increased or line length decreased. The transect width was smaller than transect spacing to avoid overlapping, but was large enough to accommodate the residual-tree spacing. For example, if the distance between trees was 11.6 m [38.1 ft] in a 74-tph [30-tpa] unit, a minimum width of 11.9 m [39 ft] was required between transects. The lines were redrawn if two transects overlapped because of narrow line spacing. All the trees were sampled within the transects.
Block along skyline corridor/ skid trail
Each skyline road or skid trail was divided into four sections. Three borderlines for each skyline road or skid trail were drawn perpendicular to the direction of the skyline/skid trail, and ran between two skyline corridors or skid trails. Each borderline served as the centerline of each block. Half the width of the block was run to the landing and the other half ran equidistant in the opposite direction. Block width was wider than residual-tree spacing. The areas of all the blocks were summed and compared with the total area originally calculated by the sample-size formula.
RESULTS AND DISCUSSION
Comparison of Damage Levels Derived from 100% Survey and Sampling Methods
For all six units, the damage estimated by each of the four sampling methods was very similar to the actual damage level determined by the 100% survey, with only a few exceptions (Table 3). The p-values were high, indicating that sampling methods provided reasonable estimates of stand damage. The ANOVA test also indicated that there was no strong evidence of differences in accuracy among the four methods (Table 4). This analysis was performed using the values of bias for each method in Table 3.
TABLE 4. Analysis of variance (ANOVA) table: a test for comparing accuracy (% bias) of stand damage estimates provided by four sampling methods.
| Source | df | Mean | F-statistic P | |
| Methods | 3 | 8.147 | 0.935 | 0.442 |
| Error | 20 | 8.716 |
The differences between actual and estimated damage were less than 2% in 12 of 24 estimates (six units by four sampling methods), including five estimates with less than 1% bias. However, two estimates from the random sampling methods were significantly different from the corresponding actual damage level (a = 0.05). Damage was also overestimated in two systematic transect sampling units, including one estimate that was significantly differed (P = 0.01).
Overall, the systematic sampling estimates appeared to be relatively consistent (-1.5 to 4.9% in bias), providing the narrowest 95% confidence intervals that included actual damage levels for all cases. With an allowable sampling error of 10% used in this study, the estimates provided by the systematic plot sampling method were highly acceptable. The standard errors for all methods ranged from 2.3 to 6.4%, creating very wide confidence intervals. For example, the 95% confidence interval for the Walk Thin site was 10.4 to 20.4% when the damage estimate from systematic plot sampling was 15.4% and the actual level was 16.9%. In sampling intensities in this study, 10 to 55% of the total unit area was sampled; the higher sampling intensity would not be practical if it was required for reducing sampling error. Bettinger and Kellogg [2] sampled about 25% of their total study area while investigating logging damage in cut-to-length thinning stands.
TABLE 3. Comparisions of damage measured in the 100% surevey to damage estimate in each of four sampline methods.
| Unit/ Logging system |
Thinning perscription (tph [tpa]) |
Sampling method |
# trees sampled |
% Damage | % Biasa | % Standard error |
p-value |
| Yachats Skyline |
247 [100] |
100% | 992 | 22.9 | |||
| Systematic | 127 | 24.4 | 1.5 | 3.8 | 0.69 | ||
| Random | 123 | 19.5 | -3.4 | 3.6 | 0.34 | ||
| transect | 146 | 23.3 | 0.4 | 3.5 | 0.91 | ||
| Block | 133 | 24.8 | 1.9 | 3.7 | 0.61 | ||
| 148 [60] |
100% | 441 | 37.4 | ||||
| Systematic | 90 | 36.7 | -0.7 | 5.1 | 0.89 | ||
| Random | 102 | 39.2 | 1.8 | 4.8 | 0.71 | ||
| transect | 95 | 30.5 | -6.9 | 4.7 | 0.14 | ||
| Block | 86 | 38.4 | 1.0 | 5.2 | 0.85 | ||
| 74 [30] |
100% | 134 | 37.3 | ||||
| Systematic | 77 | 39.0 | 1.6 | 5.6 | 0.76 | ||
| Random | 78 | 37.2 | -0.1 | 5.5 | 0.99 | ||
| transect | 84 | 35.7 | -1.6 | 5.2 | 0.76 | ||
| Block | 58 | 39.7 | 2.3 | 6.4 | 0.71 | ||
| Walk Thin Skyline |
284 [115] |
100% | 801 | 16.9 | |||
| Systematic | 201 | 15.4 | -1.4 | 2.5 | 0.55 | ||
| Random | 169 | 11.2 | -5.6 | 2.4 | 0.02 | ||
| transect | 254 | 16.5 | -0.3 | 2.3 | 0.86 | ||
| Block | 197 | 12.7 | -4.2 | 2.4 | 0.08 | ||
| Mill Thin tractor |
284 [115] |
100% | 574 | 20.6 | |||
| Systematic | 155 | 18.1 | -2.5 | 3.1 | 0.42 | ||
| Random | 175 | 15.4 | -5.1 | 2.7 | 0.05 | ||
| transect | 236 | 14.4 | -6.2 | 2.3 | 0.01 | ||
| Block | 207 | 19.8 | -0.8 | 2.8 | 0.78 | ||
| Flat Thin Cut-to- length |
284 [115] |
100% | 761 | 29.4 | |||
| Systematic | 163 | 24.5 | -4.9 | 3.4 | 0.15 | ||
| Random | 156 | 23.7 | -5.7 | 3.4 | 0.15 | ||
| transect | 272 | 27.2 | -2.2 | 2.7 | 0.42 | ||
| Block | 194 | 24.7 | -4.7 | 3.1 | 0.13 | ||
| a Bias is the difference between % damage measured in the 100% survey and % damage estimated by one of the sampling methods. Negative values indicated that sampling underestimated the level of damage. | |||||||
Ease of Layout and Field Performances for Four Sampling Methods
Systematic plot sampling
Among the sampling methods, we observed that systematic plot sampling took the least amount of time to lay out on the map and install on the site. This method also required the least physical effort to proceed in the field. In the steeply sloped skyline-logging unit, skyline roads ran perpendicular to the contour lines. The investigator, therefore, was able to walk along the contour, which eliminated climbing up and walking down slopes and avoided a slope correction for the distance between plot centers.
If reference points on the maps are available, one can check the accuracy in locating plots while sampling or can fix any deviation from the planned line. Data collection requires one person. The design samples the entire stand, with no chance for many plots to be concentrated in one area.
For parallel landings, however, care must be taken when plot spacing coincides with corridor or skid trail spacing. Damage to the remaining trees is severe along skyline corridors or skid trails, but is not frequent between them [5, 9, 15, 16].
Periodicity may occur when the spacing of plots and skyline roads or skid trails coincides. We did not experience that in this study because, although plot spacing remained the same, skyline road or skid trail spacing was not constant, even in parallel yarding, forwarding, or skidding trail units. Periodicity is not a concern in a fan-shaped or branching-skidding unit.
Alternatively, the sampling frame can be partitioned into groups or strata if an area has fan-shaped yarding or parallel skidding with different orientations. This eliminates the possibility that systematic plots will be located along skyline corridors or skid trails. Systematic plot sampling is performed independently within each stratum, but with the same probability of selection within each area (stratified systematic plot sampling). The plot estimate will be a function of data combined from individual strata. Sampling precision may be increased if plots are representative of the entire stand.
Random plot sampling
The greatest advantage in simple random plot sampling is that sampling units are chosen completely at random in the study area, with no subjectivity or bias on the part of the field sampling personnel. Every tree has non-zero probability of being selected. As in systematic plot sampling, only one person is needed for data collection. Plot layout, however, is the most complicated; it can be very difficult to locate specific plots at the site. The azimuth and horizontal distance for each plot are different, requiring a higher traversing skill and increased physical effort to climb hills. In steep terrain, a slope correction may be required.
Systematic transect
This method has advantages similar to the systematic plot sampling method, but it is difficult to check the transect width unless the centerline is marked. Compared with systematic plot sampling, the transect takes more time and effort to proceed in the field and requires greater accuracy in traversing with a hand compass. At least two persons are needed: one person to maintain a reference azimuth and the other to move within the transect area to check trees. transect width in a steep unit varies as the slope changes for slope correction, requiring frequent slope distance calculations. transects in this study run from one end of a boundary to the other, with lengths up to 912-1206 m [3000-4000 ft]. Measuring transect width is less difficult in tractor and cut-to-length logging units where slopes are gentle or flat.
Block along the skyline roads/skid trails
This method samples along skyline roads and skid trails where damage occurrence and severity are highest. Application is more practical for skyline logging than for ground-based logging because skyline roads are straight. The greatest disadvantage is that all skyline roads or skid trails must be traversed for layout. This method also consumes the most time for data collection and requires a two-person crew.
A Concept for an Easy, Quick Survey for Estimating Stand Damage
We devised this approach to provide quick monitoring during thinning operations and to determine whether the level of stand damage would be tolerable after thinning. To simplify the procedure, an assumption was made based on Han and Kellogg [9] and Bettinger and Kellogg [2]: 60% (for skyline and cut-to-length thinning) and 80% (in tractor thinning) of all damaged residual trees are located within 4.6 m [15 ft] of the centerline of skyline corridors and skid trails. An easy, quick survey method takes advantage of this concentration of stand damage. For example, one can check trees within 4.6 m [15 ft] of the centerline of a skyline corridor. The results are then extrapolated to the entire area of a logging unit.
This method is an easy and very fast procedure for monitoring logging damage. It does not require taking sample data from an entire unit, but checking only a narrow strip along one or two skyline corridors or skid trails. Only one person is needed for the survey. This is a simplified approach to manage stand damage associated with thinning operations, but further testing of the method is necessary.
CONCLUSION
All tested sampling methods provided estimates close to the 100% survey; standard errors were similar for all methods. There was no significant difference in accuracy among these methods, but estimates from systematic sampling were relatively consistent, resulting in the smallest standard deviation in its estimates. The stand was best represented by sampling over the entire area, such as with systematic plot sampling or systematic transects. For random plots, there was a chance of non-representative sampling if several plots were located within one small area of the unit. trees around landings and tailtrees were not included in block sampling to avoid bias.
Simplicity and ease of implementation was highest with systematic plot sampling, but lowest with random plot sampling and blocks along skyline corridors/skid trails. Systematic plot sampling was particularly advantageous because sampling activities could be conducted relatively easily on rough terrain. It also required the least amount of time for data collection. In random plot sampling, locating the plots on the ground was time-consuming, and a high degree of accuracy was required for pacing and following compass bearings. Map layout was simplest in the systematic transect, but it was difficult to gather data. Block sampling required that all the corridors or trails be traversed. It was difficult to apply in a branch-skidding unit because of the many short, curved trails.
Because of time and financial constraints, it is often not feasible for government agencies and private companies to conduct large-scale damage surveys after thinning operations. A simplified sampling approach is necessary to manage stand damage efficiently. An easy, quick method can provide preliminary stand damage data during harvest operations. By discussing this information with the logging contractor, one can take corrective action to prevent or reduce successive logging damage.
Acknowledgements
The authors would like to thank Steve Pilkerton and Mark Miller for assistance in making key local contacts as well as obtaining materials and supplies; and Lisa Ganio for offering useful advice on the statistical design and analysis. This research was supported by Oregon State University�s Strachan Forestry Research Fund and the USDA center for Wood Utilization.
REFERENCES
[1] Aho, P.E., G. Fiddler, and M. Srago. 1983. Logging damage
in thinned, young-growth true fir stands in California and recommendations
for prevention. USDA Forest Service, Portland, OR. Res. Pap. PNW-304. 8pp.
[Return to text]
[2] Bettinger, P. and L.D. Kellogg. 1993. Residual stand damage from
cut-to-length thinning of second-growth timber in the Cascade Range of
western Oregon. For. Prod. J. 43(11/12):59-64.
[Return to text]
[2] Bettinger, P. and L.D. Kellogg. 1993. Residual stand damage from
cut-to-length thinning of second-growth timber in the Cascade Range of
western Oregon. For. Prod. J. 43(11/12):59-64.
[Return to text]
[2] Bettinger, P. and L.D. Kellogg. 1993. Residual stand damage from
cut-to-length thinning of second-growth timber in the Cascade Range of
western Oregon. For. Prod. J. 43(11/12):59-64.
[Return to text]
[2] Bettinger, P. and L.D. Kellogg. 1993. Residual stand damage from
cut-to-length thinning of second-growth timber in the Cascade Range of
western Oregon. For. Prod. J. 43(11/12):59-64.
[Return to text]
[3] Burditt, A.L. 1981. Damage to the residual stand due to skyline
yarding. M.F. paper, Department of Forest Engineering, Oregon State University,
Corvallis. 70 pp.
[Return to text]
[4] Caccavano, M.P. 1982. Factors influencing residual stand damage
levels due to cable thinning of coniferous stands in western Oregon. M.F.
paper, Department of Forest Engineering, Oregon State University, Corvallis.
67 pp.
[Return to text]
[5] Cline, M.L., B.F. Hoffman, C. Michael, and W. Bragg. 1991. Stand
damage following whole-tree partial cutting in northern forests. North.
J. Appl. For. 8(2):72-76.
[Return to text]
[5] Cline, M.L., B.F. Hoffman, C. Michael, and W. Bragg. 1991. Stand
damage following whole-tree partial cutting in northern forests. North.
J. Appl. For. 8(2):72-76.
[Return to text]
[6] Fairweather, S.E. 1991. Damage to residual trees after cable logging
in northern hardwoods. North. J. Appl. For. 8(1):15-17.
[Return to text]
[7] Flatten, L.B. 1991. The use of small helicopter for commercial thinning
in steep, mountainous terrain. M.F. paper, Department of Forest Engineering,
Oregon State University, Corvallis. 51 pp.
[Return to text]
[8] John Balcom/Ward Northwest, Inc. 1992. traversePC, Version 2.14.
Florence, OR. USA.
[Return to text]
[9] Han, H.-S. and L.D. Kellogg. Damage characteristics in young Douglas-fir
stands from commercial thinning with four timber harvesting systems. (In press).
[Return to text]
[9] Han, H.-S. and L.D. Kellogg. Damage characteristics in young Douglas-fir
stands from commercial thinning with four timber harvesting systems. (In press).
[Return to text]
[10] Kelley, R.S. 1983. Stand damage from whole-tree harvesting in Vermont
hardwoods. J. For. 81:95-96.
[Return to text]
[11] Lanford, B.L. and B.J. Stokes. 1995. Comparison of two thinning
systems. Part 1. stand and site impacts. For. Prod. J. 45(5):74-79.
[Return to text]
[12] McLaughlin J.A. and R.E. Pulkki. 1992. Assessment of wounding at
two commercially thinned jack pine sites. North. J. Appl. For. 9(2):43-46.
[Return to text]
[13] Nyland, R.D. and W.J. Gabriel. 1972. Injuries from tree-length
skidding with rubber-tired vs. crawler tractor. State University, College
of Forestry, Syracuse University, New York. AFRI Res. Rep. No. 8. 11 pp.
[Return to text]
[14] Oregon Department Forestry. 1995. Cline basin thinning timber sale
contract No. 341-95-43. West Oregon District, Philomath, Oregon. pp. 29-30.
[Return to text]
[15] Ostrofsky, W.D., R.S. Seymour, and R.C. Lemin, Jr. 1986. Damage
to northern hardwoods from thinning using whole-tree harvesting techno-logy.
Can. J. For. Res. 16:1238-1244.
[Return to text]
[15] Ostrofsky, W.D., R.S. Seymour, and R.C. Lemin, Jr. 1986. Damage
to northern hardwoods from thinning using whole-tree harvesting techno-logy.
Can. J. For. Res. 16:1238-1244.
[Return to text]
[16] Sidle, R.C. and T.H. Laurent. 1986. Site damage from mechanized
thinning in Southeast Alaska. North. J. Appl. For. 3:94-97.
[Return to text]
[16] Sidle, R.C. and T.H. Laurent. 1986. Site damage from mechanized
thinning in Southeast Alaska. North. J. Appl. For. 3:94-97.
[Return to text]
[17] Stehman, S.V. and C.J. Davis. 1996. A general sampling strategy
for estimating residual stand damage. SUNY College of Environmental Science
and Forestry, Syracuse, New York. unpublished.
[Return to text]
[18] Thompson, S.K. 1992. Sampling. John Wiley and Sons, Inc. New York.
343 pp.
[Return to text]